This is a facilitator’s guide for The Long COVID Game, an instructional game on the increasing risks of contracting Long COVID with repeated infections.
Overview
This short (<5min) instructional game can help illustrate the risks of repeated COVID infections and the chance of acquiring Long COVID. It was designed for large groups, such as a classroom of students. The goal is to communicate the benefits of wearing a respirator, such as an N95, to reduce the number of COVID infections and thus the risk of contracting Long COVID.
Setup
Inform your audience that they will be playing a short game called The Haunted Woods. Try not to frame the exercise as a learning game or a COVID safety game; such priming can interfere with the learning outcomes. The goal is to have your audience follow the logic of the game, and then make the connection to a logical response to COVID. If you have a projector, you might help set the scene with a dark, slightly unnerving image of a forest like the one below.

Materials
Participants simply need the means to generate a random number between 1-10 to play. If they have a phone, tablet, laptop, or desktop they can use Google to generate a random number or use this shortened link: bit.ly/roll10.
Instructions
1) Read the following preface: “In a far away land, there is a village surrounded by a foreboding forest known as The Haunted Woods. All who pass through these woods are cursed, but pass through it they must. On the other side lies The Sacred Tree whose fruit has sustained the village for generations. In many cases, the curse will fade into nothing, but some are not so fortunate…”
2) Have participants generate a random number between one and ten. Ask those who rolled a two or less to raise their hand. Inform them that in this round, unfortunately their curse became permanent. Throughout the game, the hand raising acts as a compelling visual demonstration of just how many people are being permanently cursed per round.
3) Read the following passage: “The fruit sustains the villagers for awhile, but in time those who have been cursed before must make the pilgrimage again.” Have all participants generate another random number between 1 and 10. This time, ask those who rolled a three or less to raise their hands. Inform them that in this round, unfortunately their curse became permanent.
4) Read the following prompt: “Once again, the harvest sustains the villagers, but in time those who have been cursed twice before must make the pilgrimage again.”
5) Optional: At this point you can ask players if they would accept a magic talisman that would ward off the curse and prevent it from becoming permanent.
6) Have all participants generate another random number between 1 and 10. This time, ask those who rolled a four or less to raise their hands. Inform them that they are now, unfortunately, permanently cursed. If you introduced the talisman option, only those who refused its protection and rolled four or less would be permanently cursed.
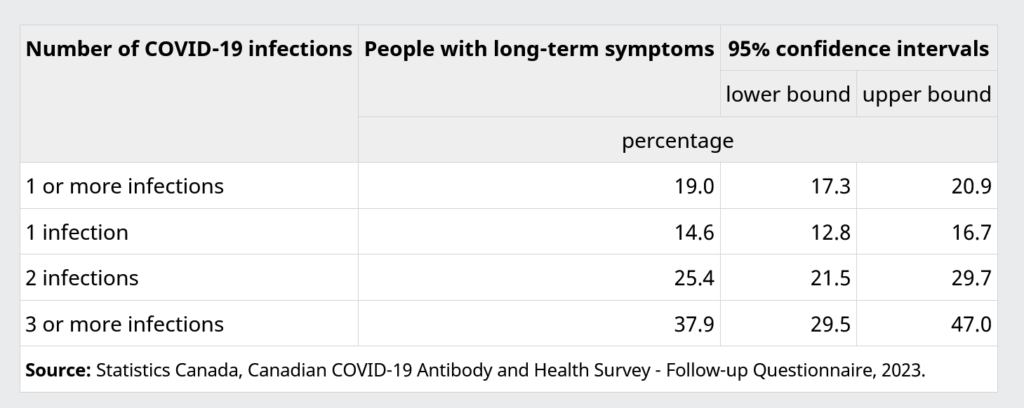
7) With the experience of the game fresh in mind, inform participants that the game simulates the percentage of people likely to have Long COVID where each pilgrimage reflects a group of people infected with COVID 1/2/3 times respectively. As reported by Statistics Canada, 15% of those who have been infected with COVID once report Long COVID symptoms; that number increases to 25% after 2 infections; 38% after 3+ infections. Note that these numbers are rounded up slightly for the game. If you gave participants the option of the talisman, draw the connection between the talisman and N95 masks, which are upwards of 98% effective at reducing the spread of COVID.1
Post-Play Debrief
The play session and reveal should be followed by a post-play debrief where you can provide additional context. I tell my students that I am not trying to alarm them, but inform them of the risks of repeated COVID infections; I make it clear that I am coming from a place of genuine concern for their well-being. I then provide the following information:


I also use the following bullet points to communicate the cognitive risks of Long COVID. As an educator, I draw attention to the dissonance between attending school to improve your thinking only to contract a disease that impairs your ability to think. At the end of the day, it just doesn’t make sense to opt out of wearing a mask.
- Mild COVID infections are associated with a drop in IQ (New England Journal of Medicine).
- In adolescents and young adults, mild COVID infections disrupt brain connectivity and reduce memory function (Translational Psychiatry)
- A single, mild COVID infection can result in the equivalent of the brain aging ten years, leading to “larger cognitive decline” compared to those uninfected (Nature)
- Mild COVID infections are associated with a drop in IQ (New England Journal of Medicine).
- “We know that people with COVID-19 are most infectious just before and as symptoms are developing, so transmission is possible before anyone knows they’re infected. Some 30% or more of infected people never develop symptoms but are still infectious.” (Center for Infectious Disease Research & Policy – University of Minnesota)
Finally, I show this short video on the science and engineering of N95 respirators. They may not be magic like the talismans, but they sure are clever and effective!
Additional Notes
Outcomes
Every audience is different and with the randomness, each iteration will play out differently. That said, since I have adopted this game I have had 98%-100% mask compliance in my classes over the past two years. I tell my university students that while one infection a year might sound reasonable, in a 4th year cohort of 100 students each infected with COVID once a year we’re likely to find 38 students with Long COVID symptoms, which could make the post-graduate process of finding work or entering grad school even more challenging than they already are.
Practical Sense
From game studies we know that players rely on what makes practical sense to them in order to play games. However, practical sense often remains situated within the context it was developed. The purpose of the post-play debrief is to connect the practical sense of the gameplay in a fictional setting with factual information on COVID, Long COVID, and mitigation strategies. For instance, the practical logic of accepting the magic talisman can reinforce the logic of wearing an N95 mask which ‘wards off’ COVID by mitigating its spread (I provide N95s for my students). This is by no means a guaranteed method of persuading your audience, just as everyone who plays Monopoly doesn’t come away seething mad about for-profit housing. But for those who aren’t receptive to being cautious with COVID, this can provide another means of challenging them to think critically about their decisions.
Design Decisions
The numbers are rounded up in the game and everyone who leaves the village gets cursed. The former keeps the numbers simple and intuitive. The latter is to reflect the risk of Long COVID at the population level where the odds increase each round, reflecting the percentage of people reporting Long COVID after 1/2/3 infections respectively. While we generally aren’t catching COVID every time we leave the house, many people have normalized at least an annual infection with this virus. If people insist on playing this game, they should at least be aware of the odds and that’s what The Haunted Forest seeks to accomplish.
Limitations
I have received a good amount of community feedback on this game over the past year, the vast majority of which has been incredibly supportive and instructive (honestly, folks have largely been great!). A subset of that feedback boils down to asking for more nuance to the game. For instance, I have focused on the reported % of people with Long COVID per number of infections in any given group. Why not focus on individual risk (see note below) and simply run the game for five or ten rounds? The short answer: time. Many of us in education are not teaching in the health sciences or science communication where a deep dive into these topics would fit with our curricular obligations; outside of those fields, we simply don’t have the luxury of carving time out of our compressed schedules to thoroughly cover the nuances of COVID and its impact on our bodies and minds. This five minute game can be integrated into most settings, while the post-play debrief can provide additional context and resources.
Change Log
1/10/2025 – Revised the guide to better distinguish from the individual risk per infection.
- Feel free to add that this population-level outcome is distinct from the probability per infection. The latter remains at 15%, while the odds accumulate with each successive infection that COVID will translate into long term complications. The game simulates the outcomes reflected in the StatsCan report where in any given group of 100 people infected with COVID 1/2/3 times we’re likely to find 15/25/38% of people with Long COVID symptoms. ↩︎
